Statistics Assignment Evaluating Different Business Scenarios
Question
Task:
Statistics AssignmentQuestion 1:
a) A researcher at a large department store finds that 30% of customers are coerced into buying an item. The researcher also finds that when coerced to buy an item, the shopper returns the item for refund 20% of the time. If a shopper is not coerced into buying an item then that item is returned 1% of the time.
i) Construct a fully labelled tree diagram to represent the above information. Hint: Firstly use C to represent the event that a shopper is coerced into buying an item and then use R to represent the event that an item is returned.
ii) Identify the branches on your tree diagram where an item is returned and use this to calculate the probability that an item is returned.
iii) Given an item is returned, calculate the probability that the shopper had been coerced into buying it.
iv) Interpret your answer in part iii) and based upon this suggest how management might use this information to guide staff sales conduct.
b) A restaurant chain routinely surveys customers regarding their dining experience and their intention to return within the next fortnight. The following table summarises the findings of the most recent survey. Answer the following questions based on these data and using appropriate notation and working.
|
Rating (Customer Experience) |
Customer will return |
Customer will not Return |
Totals |
|
Poor |
4 |
20 |
24 |
|
Fair |
16 |
18 |
34 |
|
Good |
70 |
28 |
98 |
|
Excellent |
40 |
4 |
44 |
|
Total |
130 |
70 |
200 |
i) State the proportion of customers who registered a “Good” experience and “will return” within the next fortnight?
ii) Determine the proportion of the customers who intend to return within the next fortnight?
iii) Given that a customer rated their experience as “Poor”, calculate the probability that they intend to return within the next fortnight.
iv) Considering your answers to parts ii and iii, do you think “intention to return” is independent of “customer experience”?
Explain the reasoning that led you to your conclusion.
Question 2
a) A company produces a range of snack foods, including cookies. The cookies are produced so that the weight of each cookie is normally distributed with a mean of 25g and a standard deviation of 0.8g. The cookies are then packaged in groups of 12, with an advertised net weight of 295g. Note: You may use Excel functions to answer the following questions however in order to gain full marks for a. parts ii, iii, iv and v and also b. part ii you must provide logical working that includes the Excel function, the Excel output and an appropriate diagram.
i) If X is the random variable representing the weight of a single cookie, then identify the type of distribution the random variable X has and write down the value(s) of the parameter(s) of this distribution.
ii) Determine the probability that a randomly selected cookie has a weight of less than 24.5g. (4 marks) iii) Calculate the proportion of cookies produced with weight greater than 26g.
iv) Calculate the proportion of cookies produced that have a weight within 0.5g of the population mean. v) Find the weight that 95% of cookies will exceed.
b) The quality control officer for the company is looking at the likelihood of packs of cookies being under the advertised weight. His interest therefore shifts to the mean weight of cookies in a sample of 12, which will be denoted X .
i) State the distribution of the mean of a sample of 12 randomly selected cookies (that is, X ) and give the value(s) of its parameter(s).
ii) Determine the mean weight of cookies in a pack in order for the total contents to be 295g. Hence determine the probability that a randomly selected pack of cookies has a weight that is below the advertised weight.
Question 3
A national Australian banking corporation offering services both online and through traditional branches is interested in the extent that its customers are satisfied with its services. Management accordingly hires a research firm to estimate the proportion of its Australian customers that are satisfied with the bank’s services. The research firm duly advised that 48 of a random sample of 400 of its customers indicated that they were satisfied.
a. Based upon this sample, name and give the value of the statistic that would be management’s best estimate (or a point estimate) for the proportion of the bank’s customers who are satisfied.
b. Use the Excel Estimators Macro to determine a 95% confidence interval for the proportion of the bank’s Australian customers that are satisfied. Show that the assumption/s underlying the method used are satisfied.
c. What would need to be done to get an estimate of the required population proportion that has 95% probability of being within 1 percentage point of the true value. Subsequently management finds that the research firm had in fact surveyed people (at random) as they exited a large Melbourne branch of their bank late on a Friday afternoon.
d. Comment briefly (using relevant statistical terminology) on:
i. the validity of the approach used and any implications for this inference; and
ii. whether conducting a larger survey in this same manner would likely lead to more reliable inferences.
Answer
Statistics AssignmentQuestion 1
PART A
The requisite tree diagram is shown as follows.
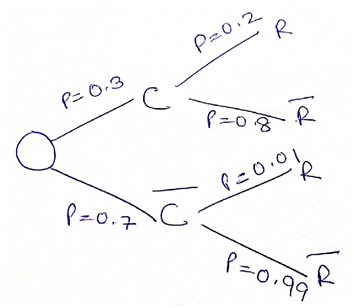
The return branches in the above decision are CR and C’R.
Probability of an item being returned = P(C and R) + P(C’ and R) = (0.3*0.2) + (0.7*0.01) = 0.67
P(C I R) = P(C and R)/P(R)
From part (ii), P(R) = 0.67
P(C and R) = 0.3*0.2 = 0.6
Hence, P(C I R) = 0.6/0.67 = 0.8955
The sales representative should try not to coerce customers and if they do, they must make sure that the customers are provided compelling reasons so as to minimise the returns.Currently, 89.55% of the returns are from coerced customers which clearly need to be addressed.
PART B
Customers who registered “Good” experience and “will return” within the next fortnight = 70
Total customers = 200
Requisite proportion = (70/200) = 7/20 = 0.35
Customers who intend to return within the next fortnight = 130
Total customers = 200
Requisite proportion = (130/200) = 13/20 = 0.65
Customers who registered “Poor” experience and “will return” within the next fortnight = 4 Total customers who registered “Poor” experience = 24 Requisite probability = (4/24) = 1/6 = 0.167
The intention to return is not independent of the customer experience. This is evident from the fact that average proportion of customers who intend to return is 0.65 while specifically for consumers who had a poor experience, the proportion significantly drops to 0.167. This is on expected lines since customers who had excellent or good experience would be more likely to return than those who had a bad experience.
Question 2
Part A
Mean weight of cookie = 25 grams
Standard deviation = 0.8 grams
Type of distribution = Normal Distribution
X ~ Normal (µ = 25, ?=0.8)
Probability (X< 24.5 grams)
P(x<24.5) = P ((x-µ)/?)< (24.5-25)/(0.8) = P (z <-0.63) = 0.2643
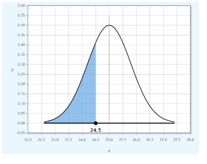
There is a 0.2643 probability that a randomly selected cookie would have mean weight less than 24.5 grams.
Proportion of cookie weight greater than 26 grams
P(x>26) = P ((x- µ)/?) > (26-25)/(0.8) = P (z > 1.25) = 0.1056
Proportion of cookie weight greater than 26 grams is 0.1056.
Proportion of cookies produced that weight within 0.5 grams of population mean
Here x1 = 25 – 0.5 = 24.5 and x2 = 25+0.5 =25.5
P(24.5 Proportion of cookies produced that weight within 0.5 grams of population mean is 0.4714. Let the required weight is c grams.
Here P(X<= c) = 0.95 Part B Probability that a randomly selected pack of cookies would have weight below the advertised weight (295 grams) P (x<= 24.583) = P (z< (24.5873 – 25)/0.2309) = 0.0351 The required probability will be 0.0351. Question 3 Point estimate p = x/n = 48/400 = 0.12
0.12 is the proportion of the bank’s Australian customers who are satisfied. Assumptions Independence assumption: The drawn sample values are independent of one other. 95% confidence interval Normal Excel View Formula Excel View 95% confidence interval = [0.088 0.152] It can be said with 95% confidence that the population proportion of bank’s Australian customers that are satisfied with the services would fall between 0.088 and 0.152.
Minimum sample size n= p (1-p) (z/E)2= I) In the given case, it is unlikely that the result obtained would be valid since the sample collected is unlikely to be representative of the underlying population. Also, the timing of the day is such that a particular type of customers would be overrepresented in the sample while others would be under-represented. This is because the appropriate sampling method should be stratified random sampling since key parameters such as age, income levels, gender may impact the satisfaction levels of the customers considering their differing expectations and preferences. The implication of this is that that the results obtained may not be reflective of the actual satisfaction levels of customers and also reliability would be lacking. II) Considering that the sample is likely to be unrepresentative of the population, hence it is unlikely that the results would significantly improve if the same methodology is used for the survey. It would be preferable to select the sample randomly from the list of all customers which are segregated as per significant parameters. Then, a telephonic or face to face survey can be held with these selected individuals which would result in better results. 
P((x-25)/0.8 < (c-25)/0.8) = 0.95
(c-25)/0.8 = 1.645
C = 26.32 grams
Sample size = 12
Type of distribution = Normal Distribution
X ~ Normal (µ = 25,?/n0.5=0.8/(12)0.5)
X ~ Normal (µ = 25, S.E. =0.2309)
Here, 2* average < = 295
Average < = 24.583
Now, 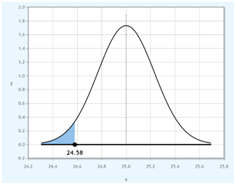
Sample proportion would be the best point estimate in this scenario.
Sample size: Here sample size is 400 which is sufficient.
Randomization condition: Here, the sample has been drawn randomly so the condition is met.
The z value for 95% confidence interval = 1.96
Lower limit = p – z ?p(1-p)/n = 0.12 – 1.96 *?0.12 (1-0.12)/400) = 0.088
Upper limit = p + z ?p(1-p)/n = 0.12 + 1.96 *?0.12 (1-0.12)/400) = 0.152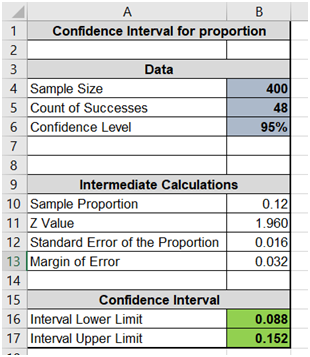
Margin of error = 1% or 0.01
Z value = 1.96
0.12*(1-0.12)*(1.96/0.01)2 = 4056.7 or 4057
Minimum sample size would be 4057 in order to get the required population proportion.