Decision Making Assignment: Marketing Mix Problem Analysis of Pedigree & Pure Pet

Question

Task: Decision Making Assignment Instructions: This is an individual assessment and you must choose one of the managerial problems that relate to your organisation or another organisation of your choice. You need to research all the data that will support management in such a way that hey will have sufficient data and information that they are able to make the decisions appropriately.

Answer

1.0 Introduction to decision making assignment
The managers’ utilisation of mathematical models has been expanded due to the advancement of computer technology and various business and engineering-based research work. Less significant details related to a system or an object is the main features of a model. With the use of various parameters and variables in the mathematical expressions, this model shows its significance in different aspects. The real system behaviours of this model vary in different conditions and this has been known by doing proper analysing and handling techniques. This allows taking necessary action and even creative systematic design (Louatiet al., 2021).

Mathematical models are far better than handling and nurturing real systems as the previous models are cheaper, safer and extremely faster. For an instant, making a paperboard from recycled scrap paper reduces the production cost. It is not deniable that a company can go through various alternations and addition regarding quality checking and cost calculation. However, lack of implementation of several combinations, the optimum combination is completely missing (Rasheed, 2019). In contrast, a mathematical model is applied to assess all the possible combinations and alterations, to pick up the best suitable one which can satisfy the product detail without any financial disruption. The trial-and-error approach is more expensive and consumes more time than mathematical modelling (Qi et al., 2021). The constrained optimisation model is responsible to formulate many tasks like product management, personnel time scheduling, vehicle routing and many other things. As a set of changing solutions, this constrained optimisation model generates the best possible solutions regarding the evaluation of some criteria (Solaja et al., 2019).

Mathematical inequalities or equalities is a set of constraints that comes under these solutions.

2.0 Background of the Problem
Two types of dog food are being manufactured by the Healthy Pet Food Company, namely Pedigree and Pure Pet. In one packet of Pedigree cereal is 2 pounds and meat is 3 pounds of meat, whereas, cereal is 3 pounds and meat is 1.5 pounds in one packet of Pure pet. The company aims to sell more of its products according to the number of dogs available. Price of one packet Pedigree is $2.80 and Pure Pet delivers the same quantity by $2.00. There are a few ways in which the production of this company is limited. It is fact that Healthy is allowed to buy cereal approximately 400,000 in one month at the cost of $0.20/pound. This company is further to allowed to buy meat in quantity around 300,000 pounds at the cost of $0.50/pound. To make Pedigree a different kind of machine is used which can manufacture around 90,000 packages in a month. The blending and packaging cost of the dog food is around $0.25 per package for Pedigree and one package of Pure Pet, costs around $0.20. This information is mentioned in the following table:

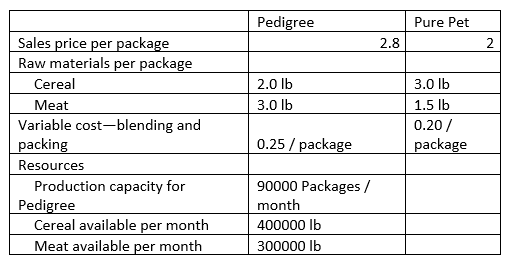
Table: Healthy Pet Food Data

2.1 Research question
It is assumed that the salary of the manager of the Dog Food Division of the Healthy Pet Food Company is determined on the basis of profit division. Therefore, it is quite true that the manager would certainly make an effort to increase the profit. Therefore, it is a questionable fact that in which way the manager would handle the division to increase the profit and his wages

3.0 The solution
3.1 The Decision Variables
In the beginning, we recognise the known variables, which is the decision variables. This problem shows that we have direct access to two quantities: the total package of Pedigree to manufacture every month and the total packages of Pure Pet to manufacture every month. In this model, both the quantities are transparent, therefore, we highlight this in a general way. Both the variables are symbolised as M and Y. M indicates the number of packages that Pure Pet manufacture in a month and Y reflects the number of packages that Pure Pet manufacture every month. It is quite true that both the variables are not good selection regarding the use of meat in a month and the use of cereals in a month. With the help of the M and Y, we initially have indirect control. Application of these variables is not fruitful, as it can lead to oracular manufacturing plans. The application of the cereals and the meats in the manufacturing issues is not may equivalent to the use of it or the overall intake of an individual dog. Moreover, calculation of the values of M and Y, allows us to recognise the production and the requirements of meat and cereals.

3.2 Objective function
The variables of M and Y is denoted as a production of manufacturing plan whenever these variables create pair of numerical values. For an instant, M=10,000 and Y=20,000 refers that the company manufactures 10,000 packets of Pedigree and 20,000 packets of Pure Pet every month. However, there is a chance of doubt whether this production plan is good or not! This problem can be overcome by the application of an objective function. The most suitable objective function is to increase the monthly revenue. (In fact, as any plan that maximises revenue minus variables cost, maximises profit simultaneously. Therefore, this is the contribution to profit as fixed costs are ignored). Each dog food made available and sold by Healthy, profits earned by the company is a direct function of the amount food sold by decision of the variables. Monthly profit as signified by z, is described below:

z = (profit per packet of Pedigree) * (number of packets of Pedigree made and sold in a month) + (profit per packet of Pure Pet) * (number of packets of Pure Pet made and sold monthly)

The profit in each package for every dog food is calculated below:

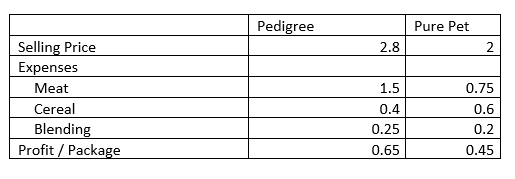
We write the month profit as
Z = 0.65 * M + 0.45 * Y

3.3 Implementation of Solution
It has been tried to maximise the value of z, so why not focus on M and y, make them infinite and infinite profit. It is not possible for the limitation on the production capacity of cereal and meat for pedigree. (Specifically, there is a limit of the demand but in here we ignore those for simplicity). The intension to get the highest of value of z, but focus is to sustaining the stated constraints. For solving those problem, it is intended to express these constraints as mathematical equalities and inequalities. Let’s start with the accessibility of cereal constraint:

(The value of lb of cereal used in manufacture each month) <= 400,000 Ib. In the left-hand side of the constraint is calculated on the basic of number of packages of pedigree and pure pet made. The L.H.S is

(lb. of cereal per packetof Pedigree) * (packets of Pedigree made and sold monthly) + (lb. of cereal per packetof Pure Pet) * (packetof Pure Pet made and sold monthly)

From each product we can substitute the cereal content and decide variables in the expression, The constraints written as
2*M + 3*Y <= 400,000
Based on same type of reasoning, the barrier on the accessibility of meat can be expressed as 3*M + 1.5*Y <= 300,000
Additionally, in these constraints, pedigree produced each month cannot cross the line of 90,000 that is, M<=90,000
Lastly, negative rate of production is senseless, so the basic of requirement M >= 0 and Y >= 0. Gathering all these in one place we get the following constrained optimization level.

Maximize z = 0.65 * M + 0.45 * Y
Subject to 2 * M + 3 * Y <= 400,000
3 * M + 1.5 * Y <= 300,000
M <= 90,000
M, Y >= 0

4.0 Excel Solver Solution
4.1 Excel Model

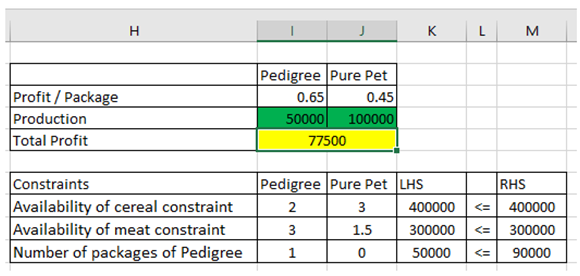
4.2 Answer Sheet

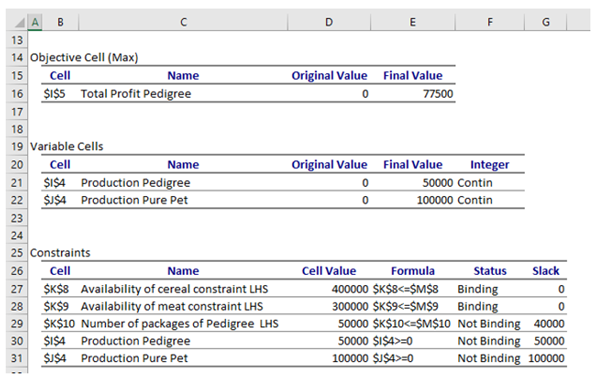
5.0 Recommendation
Linear programming model has been used to solve the problem as the function of the objective is liner and the function of all the constraints used here are linear. Let’s look at the advantages and the disadvantages of optimization models then the focus is given on the linear programming in detail. The finest result for healthy Pet problem is M = 50,000, Y = 100,000, and z = $77,500. That is, in every month healthy should product 50,000 packets of pedigree and 100,000 packets of pure pet and this production will give them $77,500 monthly profit.

6.0 Conclusion
According to the formulation linear program is a clean and simple process. In practice, optimisation models and by using linear programming is not neither static, nor straightforward. Specifically, the goal is to use the described models to derive the justified solutions that are relatively better than those who have not used any of the models. The goal is also to increase our confidence in our decisions, and to revise and update the decisions in a periodic manner.

References
Louati, A., Lahyani, R., Aldaej, A., Mellouli, R., &Nusir, M. (2021). Mixed Integer Linear Programming Models to Solve a Real-Life Vehicle Routing Problem with Pickup and Delivery. Applied Sciences, 11(20), 9551.

Qi, J., Cacchiani, V., Yang, L., Zhang, C., & Di, Z. (2021). An Integer Linear Programming model for integrated train stop planning and timetabling with time-dependent passenger demand. Decision making assignment Computers & Operations Research, 136, 105484.

Rasheed, M. S. (2019). Linear Programming for Solving Solar Cell Parameters. Insight-Electronic, 1(1).

Solaja, O., Abiodun, J., Abioro, M., Ekpudu, J., &Olasubulumi, O. (2019). Application of linear programming techniques in production planning. International Journal of Applied Operational Research-An Open Access Journal, 9(3), 11-19.