This report will explore the best strategies to calculate the Area of Equilateral Triangle. In the discipline of triangles, the triangles are the most researched and discussed ones among the mathematic researchers. In the development of geometry and other subsections of the mathematics discipline, the triangle has played a very significant and crucial role. Although the triangles are classified into many types, they are the polygons with three sides as depicted in the below figure.

According to the length of the sides and their angles, the triangle could be classified into several types. If considered the parameter of the length of the sides the triangle could be classified as an equilateral triangle, isosceles triangle, and scalene triangle. Where in equilateral triangles are the one which possesses the sides with equal length, isosceles triangles that have only its two sides equal. The triangle which possesses the sides with different or unequal length is termed to be the scalene triangle. While calculating the Area of Equilateral Triangle, the angles of the triangles also bear a great significance in geometry. The total sum of all the angles in a triangle is 180°. If considered the angles as the parameter of identification for triangles, they could be classified into acute triangles, right-angled triangles, and obtuse triangles. If any angle in the triangle is greater than 90° then the triangle could be termed as an obtuse triangle, whereas a triangle with one of the angles equal to 90°is termed as a right-angled triangle. If all the angles of a triangle are less than 90° then it is called the acute triangle. Among this, the right-angled triangle bears the largest significance since they have led to the derivation of revolutionary theorems like Pythagoras theorem leading to the birth of a new branch in mathematics called trigonometry.

If we observe our environment very cautiously, it could be understood that the triangles not only bear importance in the discipline of mathematics but also the basic structure of nature. This is because, among other geometric structures, triangles are deemed to be physically strong structures. It could be observed also practically that the metal which is shaped into two-dimensional shapes of triangles turns out to be a stronger structure than other geometrical shapes. This was confirmed by twisting the edges of the metal scraps in the different polygons which validated that the triangle is a stronger building unit.

Not only because of its strength, but triangles are also prevalent because of its geometric simplicity. The complex polygons could be analyzed and could be solved by partitioning them into simple units of triangles. In modern artforms and buildings, the complex and demanding structures are created by using different forms of triangles. For instance, if taken the case of the canopy (a curved shape) in St. Mary Axe, which is also known as Gherkin in British Museum was create using the basic building block in the shape of triangles. Hence in the virtual world, the triangle plays a very crucial role in creating a tough form of structure. Even the technology of CGI which is nowadays used in movies to technologically enhance the visual content extensively uses triangles to build very attractive structures.

In the history of mathematics, the most important and revolutionary work was The Elements that had been written by Euclid. In this book, he had done a lot of research and study on the equilateral triangles. Not even in mathematics, the discipline of physics had also tried to study the significance of equilateral triangles that was displayed in the legendary book Principia Mathematica written by Newton. The principles of the Euclid were further developed in this work and thus revealed some other undiscovered areas in mathematics.

In the history of mathematics, the most important and revolutionary work was The Elements that had been written by Euclid. In this book, he had done a lot of research and study on the equilateral triangles. Not even in mathematics, the discipline of physics had also tried to study the significance of equilateral triangles that was displayed in the legendary book Principia Mathematica written by Newton. The principles of the Euclid were further developed in this work and thus revealed some other undiscovered areas in mathematics.

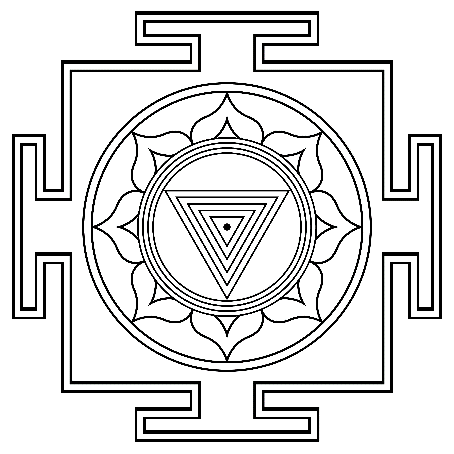
Even before their studies, the equilateral triangles were given high prominence in the archaic era that was well evident in the historical monuments like pyramids. It was between the 2613 and 2589 BC that the foremost known step pyramid was built by Snefru, one of the pharaohs of the Fourth Dynasty in Egypt. The perfect use of equilateral triangles is well evident in this structure which hence verifies that the significance of equilateral triangles was well known in the early period of the human race. The more artistic and aesthetic use of equilateral triangles were evident in the pyramids of Khufu and Giza.If looked upon the much more eastern side of the world, it could be noticed that the use of equilateral triangles was much evident in the traditions and customs followed in the tradition of Hinduism. The religion of Hinduism was indigenously generated and widely followed in the country of India. The carving in the Chennai temple and the figures used to display the Kali Yantra depict the rich and efficient use of equilateral triangles. To display the concept of eternal energy associated with the Hindu goddess, the yantra is exhibited primarily using five concentric equilateral triangles facing downwards. These five equilateral triangles were used to exhibit five tattvas that were water, earth, fire, air, and soil.

The uses of the equilateral triangles were very prevalent in ancient Chinese architecture like wooden arts. The doors were made of the wooden lattice which was carved in the form equilateral triangle to let in the air and light to the room.

Significance in the study of the area of equilateral triangle
If you are a student pursuing your degree in the discipline of mathematics, they are of equilateral triangles and are one of the prominent areas which bear great significance. Among the polygons, equilateral triangles comprise of very unique characteristics and have led to the derivation of many theories. If a student possesses a very thorough knowledge of equilateral triangles could solve any geometric problem with great ease. Although if you notice any difficulty in completing your mathematics assignment, you could approach our assignment help portal.

You should understand various components and characteristics before knowing the area of equilateral triangle. Since the equilateral triangles have all three sides equal to each other, the angles corresponding to each side are also the same which tantamount to 60 degrees.

Significant features and characteristics.
One of the major parameters to be considered about a geometric figure is its perimeter. The perimeter is the sum of the length of all the sides of the polygon. Since all the sides of the equilateral triangle are equal, you only require to multiply the length of one side with three to find the perimeter.

Although the area of equilateral triangle requires a specific methodology to be calculated since it has different geometric components and parameters. Since all the angles of the equilateral triangles are 60 degrees it would be quite clear that all the equilateral triangles are acute. (since the triangles with angles less than 90 degrees are termed to be acute-angled triangles). If led the thought process in a very reasonable way it could be observed that the equilateral triangle is also isosceles.

Methodology to calculate the area
The basic formula for calculating the area of equilateral triangle is to multiply the height of the triangle with half of the length of the base. Although this is not the only way to find the area of equilateral triangle and the methodology should be selected according to the characteristics of the triangle. There are certain formulae to find out the area of equilateral triangle and those should be selected according to the components available. The students should clarify all their doubts with their tutors before starting their geometrical assignment. If you find it very difficult to find any help in your academic institute, then you have the choice to select an assignment aiding companies available online.

If the variables are available, then the area could be calculated by using the length of base and height. The base is the length of the triangle which comes on the bottom side of the triangle, and height is considered to be the perpendicular distance from the base to the opposite point. If the equilateral triangle is right-angled then the methodology of finding the area also changes. In the right-angled triangle, the altitude of the triangle is one of the sides in the right angle and thus half of the complexity is eliminated. In the non-right-angled equilateral triangles, the student requires to derive out the perpendicular distance from the base to the opposite point. If you end up with that variable, you could easily find the area of equilateral triangle. Single is a two-dimensional figure, the area of equilateral triangle after calculating it should be represented using the square unit.

If there is a situation that you couldn’t find the altitude of the triangle, then you could also use the hero’s formula in this context. In this type of formula length of each side of the triangle is required (Although in the context the equilateral triangle, only the length of one side is required, applied that all the sides are equal). In the hero’s formula, the sum of all the sides are divided by two to calculate the semi perimeter. The student should be cautious while calculating the area of equilateral triangle using the hero’s formula since even a single mistake would make you end up with the wrong results. The hero’s formula should be implemented by following the appropriate steps and required parenthesis. The values of the length of each side of the triangle should be calculated and the results should be multiplied with each other. Then after the square root of the value is hence calculated, and thus the result is represented using a square unit.

If using another sort of method, you could use the customized equation to find the area of equilateral triangle. Since the equilateral triangle has all the sides equal, the number of variables in the equation would only be a single one. The formula is highly recommended to be used since it is the easiest way to find the value of the area. You just need to input one variable in the solution and very conveniently you could find the desirable measure.

If the variables provided don’t satisfy the above-mentioned formula, you could utilize the variables like length of two sides and the measure of the angle in between them. You should have great precision in measuring the length of the sides and the angle between those sides. By this method, the area of equilateral triangle could be calculated with great precision. If all the aspects of this approach are being implied accurately, then it is very efficient and easy to imply this method.

The possibilities are large that you would get confused about what approach to be taken to calculate the area of equilateral triangle. In such a situation, you should match the available variable and try to match it in the available formula and thus should be implied according to the convenience of the calculation. If looked at the parallelogram or other quadrilaterals, it could be observed that these polygons could be divided into basic triangles. The area of the quadrilateral could be calculated by multiplying the base with the height. The quadrilaterals could be divided into two basic triangles by using a diagonal and hence the area of equilateral triangle could be calculated using half of the base multiplied with height.

How to draw an equilateral triangle
For a student who is pursuing his degree in the discipline of mathematics, mastering the principles of geometry is the crucial step for academic accomplishment. Since the polygon of the equilateral triangle possesses the same length of sides and angles, it requires great precision and effort to draw it. Use a compass or devices to draw a perfect circle on a paper to draw an equilateral triangle. Consider a ruler with you to draw a precise straight line in a desirable measure.

By using the instruments like a compass or a ruler draw a straight line on a piece of paper. This side could be taken as one side of the equilateral triangle. The other two sides should be of the length the first line was drawn. The student has also to keep in mind that all the angles of the triangle should be 60 degrees. You should keep in mind that your first drawn straight line should only have a certain length so that the whole equilateral triangle should be enclosed in that page.

Draw an arc or segment by demarking the length of the previous straight line on the compass. TO make the segment precise and clear, please use a pencil with a sharpened edge. The arc should be drawn by pointing one of the needles of the compass at one endpoint of the previously drawn line segment. An arc is the part of the circle and it is the main component to be used in drawing the desired triangle. Your hands and fingers should be firm so that the arc should be drawn with accurate precision.

The task should be done cautiously, and it should be noted that the width adjusted in the compass should not be changed by mishandling. After drawing the first arc from one point, move cautiously the needle of the compass to another end. Draw the arc from the second point so that the second arc would coincide with the previously drawn arc. Although this task seems to be a complex one in the description, it is a very easy and enjoyable task and very less exhaustive as compared to the task of calculating the area of equilateral triangle. You should get a point where both arcs from the opposite ends of the line segment coincide. You should draw two lines from that point of coincidence to the two ends of the previously drawn line segment. These lines should be drawn using a ruler so that a perfectly straight line is obtained. When you have connected both points with the straight lines, you would end up with an equilateral triangle. After drawing the lines, delete the arcs, and you would end up with a perfect equilateral triangle.

If these apparatuses are not available, you could use an object with a circular shape or base in this process which is very convenient and easy to use. You could use the round-shaped materials like CD or a tape roll for this purpose. You could select an appropriately sized material for this purpose and could avoid any traces on the sheet. To calcite the Area of Equilateral Triangle, the initial line should be equal to the diameter of the circular object and adjusting to its radius the arc should be drawn on the paper sheet. Hence after you could create an equilateral triangle by joining the points.